Some Gaps and Examples in Intersection Theory by Fulton I
Published:
This is one of a series of blogs aiming to complete some details of the examples in this book (Intersection Theory, 2nd edition by William Fulton1) and give some comments. This blog we consider chapter 1 to chapter 6.
Part 0. Some notations
In this book we consider vector bundle as a geometric object (locally as $U\times\mathbb{A}^n$ with some cocycle condition) instead of a locally free $\mathscr{O}_X$-module, although they are equivalent: we may always let $E=\underline{\mathrm{Spec}}_X\mathrm{Sym}(\mathscr{E}^{\vee})$ where $\mathscr{E}$ be the sheaf of sections of $E$.
Chow groups in book denoted by $A_* (X)$ and here we denote it $\mathrm{CH}_* (X)$ as a more modern notation.
Here $P(E):=\underline{\mathrm{Proj}}_X\mathrm{Sym}(\mathscr{E}^{\vee})=\mathbb{P}(\mathscr{E}^{\vee})$ where $\mathscr{E}$ be the sheaf of sections of $E$. The Grassmannian $\mathrm{Grass}^e_X(\mathscr{E})$ represented by \[(f:T\to X)\mapsto\{f^* \mathscr{E}\twoheadrightarrow \mathscr{Q},\mathrm{rank}(\mathscr{Q})=e\}/\sim.\] Hence $P(E)=\mathrm{Grass}^{n-1}_X(\mathscr{E}),\mathbb{P}(\mathscr{E})=\mathrm{Grass}^1_X(\mathscr{E})$ if $\mathrm{rank}(\mathscr{E})=n$. In particuler, $\mathbb{P}^n=\mathbb{P}((\mathscr{O}_X^{n+1})^{\vee})=\mathrm{Grass}^n_X(\mathscr{O}_X^{n+1})$ and $\check{\mathbb{P}}^n:=\mathbb{P}(\mathscr{O}_X^{n+1})=\mathrm{Grass}^1_X(\mathscr{O}_X^{n+1})$. We sometimes let $\mathrm{Grass}_X(k,n):=\mathrm{Grass}_X^k(\mathscr{O}_X^n)$ and let $\mathrm{Grass}_X(k,V)$ be the $k$-planes of $V$.
As in Fulton’s B.5.7, Grassmannian in his book is also opposite to the Grothendieck’s construction. So we will use the Fulton’s notation as $G_d(E):=\mathrm{Grass}^{r-d}_X(E)\cong \mathrm{Grass}^{d}_X(E^{\vee})$ where $E$ be a vector bundle of rank $r$ on $X$.
We may let $\mathscr{O}_E(n):=\mathscr{O}_{P(E)}(n)$.
Chapter 1. Rational Equivalence
Section 1.6. Alternate Definition of Rational Equivalence
Example 1.6.3.
Section 1.9. Affine Bundles
Example 1.9.1. This construction is called affine stratification! The conclusion can be eaily deduced byinduction, Proposition 1.8 and $\mathrm{CH}_i(\mathbb{A}^n)$. For details we refer [3264]2 section 1.3.5. $\blacksquare$
Chapter 2. Divisors
Section 2.6. Gysin Map for Divisors
Example 2.6.3.(a) Let $L$ be a line bundle over $X$ with complement of zero section $L-\{0\}$. For $k\geq 0$, we of course get 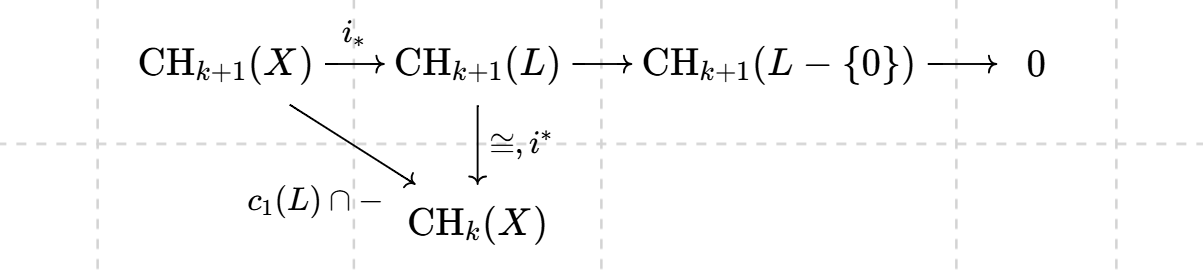
as after checking the cocycle condition, we get the normal bundle $N_{X/L}\cong L$ and by Proposition 2.6(d) we get $i^* i_* \alpha=c_1(L)\cap\alpha$. $\blacksquare$
(b) Here $X\subset\mathbb{P}^n$ with affine cone $V\subset\mathbb{A}^{n+1}$. I think here $V$ be the pointed affine cone: since we have $X\cong\underline{\mathrm{Proj}}_X\mathrm{Sym}(\mathscr{O}(1))$, the affine cone is $\underline{\mathrm{Spec}}_X\mathrm{Sym}(\mathscr{O}(1))$ and the pointed affine cone is $\underline{\mathrm{Spec}}_X\mathrm{Sym}(\mathscr{O}(1))-\{0\}$. $\blacksquare$
Chapter 3. Vector Bundles and Chern Classes
Section 3.2. Chern Classes
Example 3.2.7. For vector bundles $E,F$ over $X$ of rank $r,s$, we defined $c(F-E):=c(F)/c(E)=c(F)s(E)=\cdots$. We claim that \[c_{s-r+1}(F-E)\cap\alpha=p_* (c_s(p^* F\otimes\mathscr{O}_E(1))\cap p^* \alpha)\] where $\alpha\in\mathrm{CH}_* (X)$ and $p:P(E)\to X$. Actually we have
\begin{align*} &c_{s-r+1}(F-E)\cap\alpha\\ &=\sum_{i+j=s-r+1}c_i(F)s_j(E)\cap\alpha\\ &=\sum_{i+j=s-r+1}c_i(F)p_*(c_1(\mathscr{O}_E(1))^{r-1+j}\cap p^*\alpha)\\ &=\sum_{s-i=r-1+j}c_i(F)p_*(c_1(\mathscr{O}_E(1))^{r-1+j}\cap p^*\alpha)\\ &=p_*\left(\sum_{s-i=r-1+j}c_i(p^*F)c_1(\mathscr{O}_E(1))^{r-1+j}\cap p^*\alpha\right)\\ &=p_*\left(\sum_{i=0}^sc_{s-i}(p^*F)c_1(\mathscr{O}_E(1))^i\cap p^*\alpha\right)\\ &=p_*\left(c_s(p^*F\otimes\mathscr{O}_E(1))\cap p^*\alpha\right). \end{align*}
Hence well done. $\blacksquare$
Example 3.2.13. Here Fulton define the Euler characteristic as $\int_X c_n(T_X)$, we need to verify that this is coincident with the original one when $X$ is projective smooth variety over $\mathbb{C}$!
By Example 3.2.5 (Borel-Serre identity), we get \[c_n(T_X)=\mathrm{td}(T_X)\sum_{p=0}^n(-1)^p\mathrm{ch}(\bigwedge^p\Omega_X).\] Use Hirzebruch-Riemann-Roch theorem (Corollary 15.2.1) to $\sum_{p=0}^n(-1)^p\bigwedge^p\Omega_X$ we get \[\int_Xc_n(T_X)=\sum_{p,q}(-1)^{p+q}h^p(X,\bigwedge^q\Omega_X).\] By Hodge decomposition theorem we get \[\chi(X)=\sum_{r}{(-1)}^rh^r(X(\mathbb{C}),\mathbb{C})=\sum_{p,q}(-1)^{p+q}h^p(X,\bigwedge^q\Omega_X)\] and well done. $\blacksquare$
Example 3.2.19.
Example 3.2.22. We have $\mathrm{Hilb}_{\mathbb{P}^3}^P=\check{\mathbb{P}}^3$ where $P$ be the Hilbert polynomial of 2-planes in $\mathbb{P}^3$. Hence we get \[0\to S\to\mathscr{O}_{\check{\mathbb{P}}^3}^{\oplus 4}\to Q\cong\mathscr{O}_{\check{\mathbb{P}}^3}(1)\to0.\] Hence let $E:=\mathrm{Sym}^2(S^{\vee}\otimes Q^{\vee})$ and consider $P(E)$. Hence the variety of conics meeting a given line is given by the vanishing of a section of $\mathscr{O}_E(1)$.
Let $h=c_1(Q)$, we get $1=c(\mathscr{O}_{\check{\mathbb{P}}^3}^{\oplus 4})=c(S)(1+h)$ and hence $c(S)=1-h+h^2-h^3$. Hence we get \[c(S^{\vee}\otimes Q^{\vee})=\sum_{i=0}^3(1-h)^{3-i}c_i(S^{\vee})=1-2h+2h^2.\] Finally, if $\mathrm{rank}(F)=3$, we have \[c(\mathrm{Sym}^2(F))=1+4c_1+5c_1^2+5c_2+2c_1^3+11c_1c_2+7c_3.\] Hence we get \[c(E)=1-8h+30h^3-60h^3.\] Hence \[s(E)=\frac{1}{1-8h+30h^3-60h^3}=1+8h+34h^2+92h^3.\] Let $p:P(E)\to\check{\mathbb{P}}^3$. Hence
\begin{align*} &\int_{P(E)}c_1(\mathscr{O}_E(1))^8\cap [P(E)]\\ &=\int_{\check{\mathbb{P}}^3}p_* (c_1(\mathscr{O}_E(1))^8\cap p^* [\check{\mathbb{P}}^3])\\ &=\int_{\check{\mathbb{P}}^3} s_3(E)=92. \end{align*}
Others are the same.
Section 3.3. Rational Equivalence on Bundles
Example 3.3.2. Now we get $q:P(E\oplus 1)\to X$ and $j:E\to P(E\oplus 1)$ and the universal exact sequence \[0\to\mathscr{O}_{E\oplus 1}(-1)\to q^* (E\oplus 1)\to \xi\to 0.\] Hence we get $s^* s_* (\alpha)=q_* (c_r(\xi)\cap\bar{s}_* (\alpha))$ where $\bar{s}=j\circ s$. Hence we have
\begin{align*} &q_* (c_r(\xi)\cap\bar{s}_* (\alpha))\\ &=\sum_{i=0}^r q_* (c_1(\mathscr{O}_{E\oplus 1}(1))^ic_{r-i}(q^*E)\cap\bar{s}_* (\alpha))\\ &=\sum_{i=0}^r c_{r-i}(E)q_* (c_1(\mathscr{O}_{E\oplus 1}(1))^i\cap\bar{s}_* (\alpha))\\ &=c_r(E)\cap\alpha+\sum_{i=1}^r c_{r-i}(E)q_* (c_1(\mathscr{O}_{E\oplus 1}(1))^i\cap\bar{s}_* (\alpha))\\ &=c_r(E)\cap\alpha \end{align*}
since $c_1(\mathscr{O}_{E\oplus 1}(1))^i\cap\bar{s}_* (\alpha)=0$ by definition of $\bar{s}$. $\blacksquare$
Chapter 4. Cones and Segre Classes
Section 4.1. Segre Class of a Cone
Example 4.1.4. (Grassmann–Plücker relations). Here $X=\mathbb{A}^{mn}$ where $m\geq n$ with coordinates $(x_{ij})$ and $X$ be the locus of rank $<n$ with ideal sheaf $I$ (generated by all $n$-minors of $(x_{ij})$). Let $C=C_XY$. For any $(i)=(i_1,…,i_n)$ where $1\leq i_1<\cdots<i_n\leq m$, let $t_{(i)}$ be a variable and $\delta_{(i)}$ be the corresponding minor of $(x_{ij})$. Now consider the embedding \[P(C)=\underline{\mathrm{Proj}}_X\bigoplus_{n\geq 0}I^n/I^{n+1}\to X\times\mathbb{P}^N\] given by surjection $\mathrm{Sym}\mathscr{O}_X^{N+1}\to \bigoplus_{n\geq 0}I^n/I^{n+1}$ as $t_{(i)}\mapsto\delta_{(i)}$ where $N=\binom{m}{n}-1$.
What is Plücker relations? As we all know we have the closed embedding $\mathrm{Grass}(k,n)\to\mathbb{P}^{\binom{n}{k}-1}$ given by $\mathrm{span}(w_1,…,w_k)\mapsto[w_1\wedge\cdots\wedge w_k]$ (more generally, we have $\mathrm{Grass}^e(\mathscr{E})\to\mathbb{P}\left(\bigwedge^e\mathscr{E}\right)$). Actually the Plücker relations are just the things generated the ideal sheaf of $\mathrm{Grass}(k,n)$ in $\mathbb{P}^{\binom{n}{k}-1}$. In the special case, let $W’$ be the $k$-dimensional subspace spanned by the basis of column vectors $W_1,…,W_k$. Let $W$ be the $n\times k$ matrix of homogeneous coordinates, whose columns are $W_1,…,W_k$. For any ordered sequence $1\leq i_1<\cdots< i_k\leq n$ of $k$ integers, let $W_{i_1…i_k}$ be the determinant of the $k\times k$ matrix whose rows are the $i_1,…,i_k$ rows of $W$. Then, up to projectivization, $\{W_{i_1…i_k}\}$ are the Plücker coordinates of the element $W’$ of the Grassmannian. One can show that the embedding determined by: for any $i_1<\cdots< i_{k-1}$ and $j_1<\cdots< j_{k+1}$ in $[1,n]$, we have \[\sum_{l=1}^{k+1}(-1)^lW_{i_1…i_{k-1}j_l}W_{i_1…\hat{j}_l…i_{k+1}}=0.\]
Back to our example. Hence by definition we have embeddings \[P(C)\to X\times\mathrm{Grass}_{k}(n,m)\to\mathbb{P}^{\binom{m}{n}-1}\] and $P(C)=\{(\phi,L):\mathrm{Im}(\phi)\subset L\}$. $\blacksquare$
Section 4.2. Segre Class of a Subscheme
Example 4.2.9. (Local Euler obstruction and Nash blow-up). What is Nash blow-up? For a variety $X$ of dimension $r$ with an embedding $X\to Y$ for a non-singuler variety of dimension $n$. Consider the canonical map \[\tau:X^{\mathrm{reg}}\to X\times\mathrm{G}_r(TY),x\mapsto(x,T_{X,x})\] and let $X’:=\overline{\mathrm{Im}\tau}$. Then $\nu:X’\to X$ is called the Nash blow-up of $X$. The idea of this is that we can replace each singular point by all limiting positions of the tangent spaces at the non-singular points. (1. Nash blowing-up is locally a monoidal transformation; 2. Although the above construction uses an embedding, the Nash blow-up itself is unique up to unique isomorphism.) The more result of this and local Euler obstruction, one can read Note on MacPherson’s local Euler obstruction. $\blacksquare$
Section 4.3. Multiplicity Along a Subvariety
Example 4.3.2. (Symmetric product of non-singular curves). Fix a non-singular projective curve $C$ over some algebraically closed field $k$. We consider $C^d=C\times\cdots\times C$ with a canonical $C^{(d)}:=\mathfrak{S}_d$-action. By D. Mumford’s theory, the scheme (coarse moduli space) $C^d/\mathfrak{S}_d$ exists. One can show that $C^{(d)}$ is nonsingular for all $d$ (see Milne-Jacobian Proposition 3.2. In SGA-I, purity theorem says that $V/G$ can be nonsingular only if the ramification locus is empty or has pure codimension $1$ in $V$ . This implies that $V/G$ can be nonsingular only if $V$ is a curve!) Milne also shows that $C^{(d)}$ is the fine moduli space of degree $d$-(relative) effective divisors, hence $C^{(d)}\cong\mathrm{Hilb}^d_{C/k}$. More properties of $C^{(d)}\to \mathrm{Jac}(C)$ we refer A-V Theorem 25.1.
Now let $\deg D=d,\dim|D|=r$, we claim that $s(|D|,C^{(d)})=(1+h)^{g-d+r}\cap[|D|]$. For $d$ large, the map $u_d:C^{(d)}\to \mathrm{Jac}(C)$ is a projective bundle $P(E)$ where $E$ is a vector bundle of rank $d+1-g$ over $\mathrm{Jac}(C)$. Hence $r=d-g$. In this case $N_{C^{(d)}}(|D|)$ is tirvial, hence $s(|D|,C^{(d)})=[|D|]$ which proves the claim. For small $d$ consider $C^{(d)}\subset C^{(d+s)},E\mapsto E+sP_0$. By some simple analysis (see Theorem 2 in section 3 in paper [Schwarzenberger]3, he showed that $i_d:C^{(d)}\subset C^{(d+1)}$ as a divisor correspond to $\mathscr{O}_{C^{(d+1)}}(1)$ such that $i_d^* \mathscr{O}_{C^{(d+1)}}(1)=\mathscr{O}_{C^{(d)}}(1)$) we find that the normal bundle on $|D|$ has Chern class $(1+h)^s$. Well done. $\blacksquare$
Section 4.4. Linear Systems
Example 4.4.3. Let $X$ be an irreducible hypersurface $V_+(F(X_0,…,X_{n+1}))\subset\mathbb{P}^{n+1}$. The singular locus $J\subset X$ defined by $(\frac{\partial F}{\partial X_0},…,\frac{\partial F}{\partial X_{n+1}})$. Taking blowing up $\pi:X’:=\mathrm{Bl}_J X\to X$ with $f:X’\to\mathbb{P}^{n+1}=P(V^{\vee})$ where \[V=\mathrm{Span}\left\{\frac{\partial F}{\partial X_0},…,\frac{\partial F}{\partial X_{n+1}}\right\}\subset H^0(X,\mathscr{O}_X(d-1))\] be a linear system of $X$ with base locus $J$. Define $X^{\vee}:=f(X’)\subset P(V^{\vee})$ be the dual variety of $X$. Hence we have
\begin{align*} \deg f_* [X']&=\int_Xc_1(\mathscr{O}(d-1))^n-\int_Jc(\mathscr{O}(d-1))^n\cap S(J,X)\\ &=(d-1)^n\int_Xc_1(\mathscr{O}(1))^n-\int_J(1+c_1(\mathscr{O}(d-1)))^n\cap S(J,X)\\ &=(d-1)^n\deg X-\sum_{i=0}^n\binom{n}{i}\int_Jc_1(\mathscr{O}(d-1))^i\cap S(J,X)\\ &=d(d-1)^n-\sum_{i=0}^n\binom{n}{i}(d-1)^i\int_Jc_1(\mathscr{O}(1))^i\cap S(J,X)\\ &=d(d-1)^n-\sum_{i=0}^n\binom{n}{i}(d-1)^i\deg(s_i), \end{align*}
where $s_i$ be the $i$-component of $S(J,X)$. $\blacksquare$
Chapter 5. Deformation to the Normal Cone
No gaps.
Chapter 6. Intersection Products
Section 6.1. The Basic Construction
Example 6.1.2. See the answer in Calculating the distinguished varieties of intersection product. $\blacksquare$
Example 6.1.4.
Section 6.3. Excess Intersection Formula
Example 6.3.4. After some reduction, we let $\alpha=[V],V=Y’$ and $i^!\alpha=s_E^!\alpha=s_{f^* E}^!\alpha$. We need to show that \[j_* s_{f^* E}^![Y’]=c_d(f^* E)\cap [Y’].\]
Example 6.3.5.
[FulIT2nd] William Fulton. Intersection Theory, 2nd. Springer New York, NY. 1998. ↩
[3264] David Eisenbud and Joe Harris, Harvard Unive 3264 and All That: A Second Course in Algebraic Geometry. Cambridge University Press, 2016. ↩
[Schwarzenberger] Schwarzenberger. Jacobians and symmetric products[J]. Illinois Journal of Mathematics, 1963, 7(2): 257-268. ↩